Ternary Phase Diagrams

EENS 2120	Petrology
Tulane University	Prof. Stephen A. Nelson
Ternary Phase Diagrams

Crystallization in Ternary Systems

I. Equilibrium Crystallization Where all 2 Component Systems are Binary Eutectic Systems.

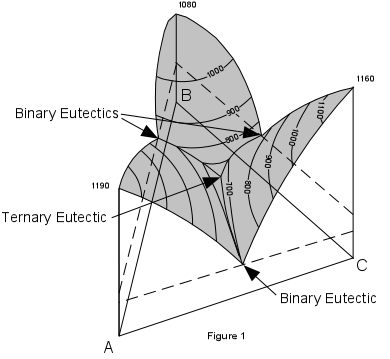
Figure 1 shows a three dimensional representation of the three component (ternary) system ABC. Note that composition is measured along the sides of the basal triangle and temperature (or pressure) is measured vertically. The top of the figure shows a surface with contour's representing lines of constant temperature. These contours are called isotherms. Note that the eutectic points in each of the binary systems project into the ternary systems as curves. These curves are called boundary curves, and any composition on one of these curves will crystallize the two phases on either side of the curve.

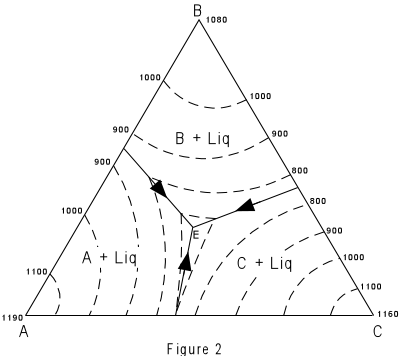
Figure 2 shows the same figure in two dimensions as seen from above. The boundary curves and isotherms are also shown projected onto the basal triangle. Note how the temperature decreases toward the center of the diagram

In Figure 3 we trace the crystallization of composition X. Figure 3 is the same as Figure 2, with the isotherms left off for greater clarity.

Note that the final solid must consist of crystals A + B + C since the initial composition is in the triangle ABC.

At a temperature of about 980° the liquid of composition X would intersect the liquidus surface. At this point it would begin to precipitate crystals of C. As temperature is lowered, crystals of C would continue to precipitate, and the composition of the liquid would move along a straight line away from C. This is because C is precipitating and the liquid is becoming impoverished in C and enriched in the components A + B.

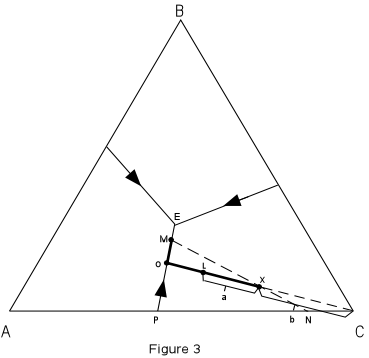At a temperature of about 820°, point L in Figure 3, we can determine the relative proportion of crystals and liquid.

% crystals = a/(a+b)*100

% liquid = b/(a+b)*100

With further cooling, the path of the liquid composition will intersect the boundary curve at point 0. At the boundary curve crystals of A will then precipitate. The liquid path will then follow the boundary curve towards point M. The bulk composition of the solid phase precipitated during this interval will be a mixture of A + C in the proportion shown by point P.

At point M, the bulk composition of the solid phases so far precipitated through the cooling history lies at point N (the extension of the straight line from M through the initial composition X). At this time the % solid will be given by the distances :

(distanceMX/distanceMN)*100

and the % liquid by the distances:

(distanceXN/distanceMN)*100

Note, however, that the solid at this point consists of crystals of A and crystals of C. So, we must further break down the percentages of the solid. This is done as follows: The percentage of the solid that is A will be given by the distance from C to N relative to the distance between A and C; i.e. by the formula:

%A in solid = (distanceNC/distanceAC)*100

Similarly, the percentage of the solid consisting of crystals of C is given by the formula:

%C in solid = (distanceAN/distanceAC)*100

We can now calculate the exact percentage of all phases present in composition X at a temperature of 660° (where the liquid composition is at point M). The following formulae apply:

%A crystals = %A in the solid * %crystals/100

%A = (distanceNC/distanceAC)*100 * (distanceMX/distanceMN)

and

%C crystals = %C in the solid * % crystals/100

%C = (distanceNA/distanceAC)*100 * distanceMX/distanceMN

Note also that we can determine the composition of all phases present in the system at this point. The composition of the liquid is given by reading the composition of point M off the basal triangle. Since it is a mixture of A, B, and C, it will have a composition expressed in terms of the percentages of A, B, and C. The composition of the solids are 100%A and 100% C; i.e. they are pure solid phases (not mixtures).

With further cooling, the liquid composition will move to the ternary eutectic, E, at a Temperature of about 650°, at which point crystals of B will precipitate. The temperature will remain constant until all of the liquid is used up. The final crystalline product will consist of crystals of A + B + C in the proportions given by the initial composition X.

Crystallization will proceed in an analogous manner for all other compositions in the ternary system.

To summarize, we can express the path of crystallization for composition X in an abbreviated form as follows:

T > 980° All Liquid

980°-680° Liq + C

680°-650° Liq + C + A

T = 650° Liq + C + A + B

T < 650° C+ A + B (all solid)

At any temperature an isothermal plane can be constructed through the system that will show the phases present for all compositions in the ternary system. Such an isothermal plane for the system ABC at 700° is shown in Figure 4.

II. Crystallization in Ternary Systems that Contain a Compound that Melts Congruently.

A ternary system that has a binary system with a compound that shows congruent melting (melts to a liquid of its own composition) is shown in Figure 5. Also shown is the binary system X-Y that contains the intermediate compound W. The result of the addition of this intermediate compound is essentially that the ternary system XYZ is divided into two smaller ternary systems represented by triangles WYZ and XWZ.

Crystallization in this system is illustrated in Figure 6, where the isotherms have been removed for simplicity.

We first note that any composition within the triangle WYZ must end up with crystals of W + Y + Z in the final crystalline product, compositions in the triangle XWY will end up with crystals of X + W + Y, and compositions on the line WZ must end up with crystals of W + Z only.

Consider first crystallization of composition A in Figure 6. Crystallization begins at about 1160° with separation of crystals of Z. The composition of the liquid then changes along a straight line away from Z. When the temperature reaches about 680°, the liquid composition has intersected the boundary curve at point B.

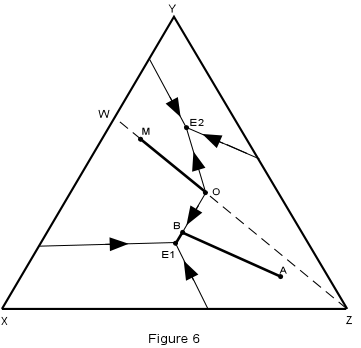At this time, crystals of W begin to separate and with further lowering of temperature, the liquid moves along the boundary curve, B-E1, precipitating crystals of Z + W. When the liquid reaches the ternary eutectic, E1, crystals of X begin to separate along with crystals of W and Z. The temperature remains constant at 640° until all of the liquid is used up leaving a final product of crystals of X + W + Z in the proportions of the original composition, A.

We can summarize this crystallization history in abbreviated form as follows:

T > 1160° All liquid

1160°-680° Liq + Z

680°-640° Liq + Z + W

T = 640° Liq + Z + W + X

T < 640° Z + W + X (all solid)

Now consider the crystallization of composition M which lies on the binary system W-Z. Since this is a binary system, only phases W and Z will be found in the final crystalline product. Thus, crystallization will stop when the liquid composition reaches the point O, which is equivalent to the binary eutectic in the system W-Z.

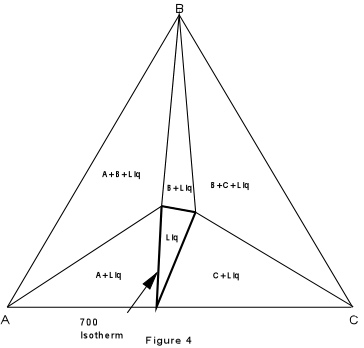
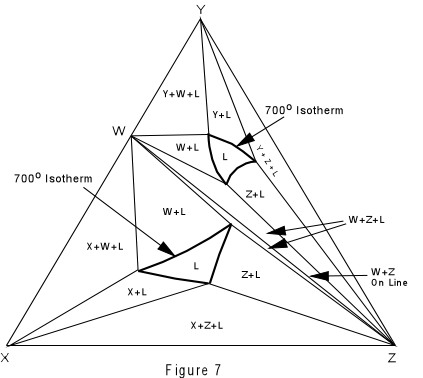
Again, we can construct isothermal planes showing the phases present in any part of the system at any temperature of interest. Such an isothermal plane at 700° for the system XYZ is shown in Figure 7.

III. Crystallization in Ternary Systems Containing an Incongruently Melting Compound.

A. Equilibrium Crystallization

Figure 8 shows the ternary system ABC and one of the binary systems AB that contains a compound, D, that melts incongruently. Note that incongruent melting of D continues into the ternary system.

We will consider equilibrium crystallization of compositions P, Q, S, T and X, as all will behave somewhat differently.

Crystallization of composition P

Since composition P lies in the triangle ADC, it must end up with crystals of A + D + C in its final crystalline product. As the temperature is lowered, at about 1090°, crystals of A will begin to precipitate. With continued cooling, the composition of the liquid will change along the line away from A toward T, meanwhile, crystals of A continue to separate.

When the temperature reaches 800°, at point T, crystals of D will precipitate along with crystals of A.

With further cooling, the liquid will change composition along the boundary curve toward R while the liquid reacts with crystal of A to produce crystals of D. Finally when the temperature reaches about 680° at point R, crystals of C will begin to precipitate while the liquid would react with some of the crystals of A until all the liquid is used up. Crystallization would then cease, and temperature would drop below 680°, leaving a mixture of crystals of A + D + C in the original proportion of composition P.
Composition Q, which lies on the join between D and C must end up with only crystals of D + C. Crystallization of Q would follow a similar path as that of composition P, first precipitating crystals of A from Q to T, then liquid reacting with A to make D while the liquid composition moves along the boundary curve to R. At R, all of the previously precipitated A would be used up to make crystals of D, and crystals of C would separate.

Crystallization would cease at point R leaving crystals of D + C in the original proportion as composition Q.
We next consider crystallization of composition S which lies in the triangle DBC. Thus, complete crystallization of composition S will result in a final assemblage of crystals of D + B + C. Crystallization begins at a temperature of about 850° with separation of crystals of A. This continues as the liquid composition changes from S to T as the temperature drops to 800°. At 800° (T) the liquid begins to react with crystals of A to form crystals of D. The liquid composition then changes along the boundary curve towards point U as the temperature is reduced further. By the time point U is reached, all of the crystals of A have been remade to crystals of D. The composition of the solid phase precipitating is now pure D, as indicated by the line from the liquid composition at U through the initial composition S. The liquid path then leaves the boundary curve at U and moves across the field where pure D is crystallizing. When the liquid composition reaches point N on the boundary curve between D + LIQ and C + LIQ, crystals of C begin to form. The liquid then moves along the boundary curve toward the eutectic, E. At E, crystals of B begin to separate and the temperature remains constant until all of the liquid is gone. The final assemblage consists of crystals of B + D + C in the proportion of the original composition.

Note that all compositions in the triangle DBC will reach the ternary eutectic, E, while those compositions in the triangle ADC will reach only the ternary peritectic, R.

Note also that a composition, such as T will leave a boundary curve when the line through the liquid composition and the original composition intersects the intermediate compound, D. This occurs almost immediately after crystallization begins. The path of crystallization for composition T is listed below:

T > 800° All Liquid

T = 800° Liq + D + A

800°-690° Liq + D

690°-580° Liq + D + B

T = 580° Liq + D + B + C

T < 580° D + B + C

The crystallization path of the composition x is shown in abbreviated form below.

T > 860° All Liquid

860°-810° Liq + D

810°-580° Liq + D + B

T = 580° Liq + D + B + C

T < 580° D + B + C

B. Fractional Crystallization

We next consider what might happen under conditions of fractional crystallization in the system. As you recall, fractional crystallization occurs when a crystalline phase is somehow removed from the system and is thus prevented from reacting with the liquid to form different crystals.

Consider now fractional crystallization of composition P in Figure 8. Under equilibrium conditions, composition P would follow the path discussed above, becoming completely solid at the ternary peritectic, R, with an assemblage of crystals of A + D + C.

We will consider fractional crystallization in steps for this example. That is at various points we will imagine all of the previously precipitated crystals are somehow removed. At 1090° composition P begins to crystallize. Crystals of A separate from the liquid. On cooling to point Q, we remove all of the previously precipitated crystals of A, our system now has composition Q, since we have removed part of the system that has already crystallized as crystals of A.

Cooling to point S, more crystals of A precipitate, and again are removed from the system. Note that at this point, the system has the composition S. Further cooling of composition S, without removing any more crystals from the system, would result in the liquid composition following the equilibrium crystallization path of composition S, as discussed above. Note that composition S is in the triangle D-C-B, and would end up crystallizing D, C, and B, in contrast to the assemblage that would have crystallized from composition P (A, D, and C) if it had crystallized under equilibrium conditions. Thus fractional crystallization of composition P would result in not only a different final crystalline assemblage, but a final liquid composition which is very different from the final liquid composition that would result from equilibrium crystallization.

If we continue our fractional crystallization of composition P, starting with the liquid (and system) having a composition of S, further cooling to point T on the boundary curve, results in further precipitation of A. If we remove all crystals of A, the system now has a composition T. Because no crystals are present to react with the liquid to form crystals of D, the liquid composition will not change along the boundary curve towards R, but instead will move directly across the field where only D is precipitated. When the temperature reaches 680°, at point V, crystals of B form and the liquid composition changes along the boundary curve toward E. At E, crystals of C form and our final assemblage consists of crystals of D + B + C. Note how fractional crystallization has allowed the liquid to become enriched in B, while under equilibrium conditions no crystals of B could have formed.

The system discussed above is very similar to the behavior of the system Mg₂SiO₄ - SiO₂ - CaAl₂Si₂O₈, where A = Forsterite, B = Quartz, C = Anorthite, and D = Enstatite. Thus, knowledge of the crystallization behavior of such a system is in many ways analogous to what may happen in magmas and shows how a basaltic magma, that would normally only crystallize forsterite, enstatite, and plagioclase, could change to a rhyolitic magma that would crystallize quartz.

IV. Ternary System with a Binary Solid Solution.

Figure 9 shows the ternary system Albite - Anorthite - Diopside. As you recall, albite and anorthite form a complete solid solution series (the plagioclase series). Anorthite and diopside form a eutectic system, as do albite and diopside. However, the eutectic in the system albite-diopside is very close to pure albite. The solid solution between albite and anorthite continues into the ternary system and is expressed by the boundary curve connecting the two binary eutectics. Note that this boundary curve forms a "temperature valley" in the ternary system.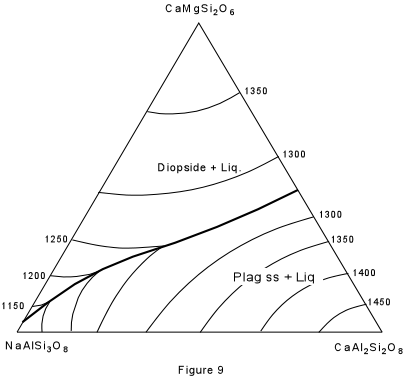

We will now consider equilibrium crystallization of 2 compositions on the plagioclase side of the boundary curve. It must be noted that geometrical methods will not predict the exact path of equilibrium crystallization in systems of this type. We here give an approximate path consistent with experimental results.

First, consider crystallization of composition D, in Figure 10, which is 27% Albite, 46% Anorthite and 28% diopside. The final product should consist of pure diopside and a plagioclase of composition 63% Anorthite.

At a temperature of about 1325° the liquid begins to crystallize with separation of plagioclase of composition 99% anorthite. As the liquid is cooled, it moves along the curved path D-P, while continually reacting with the previously precipitated plagioclase to form a more albitic plagioclase.

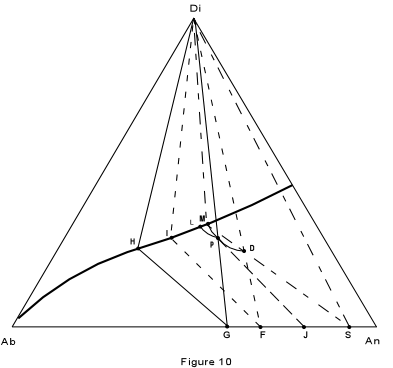By the time the liquid composition has reached point P, the Plagioclase has the composition 98% anorthite. this is found by extending a line from the liquid composition through the initial composition (D) back to the base of the triangle.

With continued cooling, the liquid composition will eventually reach the boundary curve at point M, at which time the plagioclase has the composition S (90% anorthite).

We now construct what is known as a three phase triangle. It is shown in the figure by the straight line connecting the three phases in equilibrium, Di (solid), liquid (point M) and plagioclase solid solution (Point S).

With continued cooling, the liquid composition changes along the boundary curve towards pure albite. Meanwhile the plagioclase solid solution is continually made over to more albitic compositions.

Crystallization ceases when the base of the three phase triangle intersects the original liquid composition, D. Such a three phase triangle with apices Di, I, F, is shown in the figure. It indicates that the last liquid has the composition I, and is in equilibrium with pure diopside and a plagioclase composition of 70% anorthite (point F).

Crystallization of composition P will be similar to that of D. Note, however that the liquid composition will follow a different path and intersect the boundary curve at point L. The liquid composition will then change along the boundary curve until the base of the three phase triangle Di, H, G, intersects the initial composition P. The final assemblage will then consist of pure diopside and plagioclase of composition G (60% anorthite).

We now consider what would happen if the solid material were removed somehow, and prevented from reacting with the liquid. This is the case of fractional crystallization. Suppose we start again with composition D and cool it to a point where the liquid has the composition P. If we now remove all of the plagioclase that has crystallized up to this point, our liquid, and thus our entire system (without the removed crystals) now has the composition P. As we saw in the example above, composition P follows a different path of crystallization than composition D, and will produce a plagioclase of more albitic composition that of composition D. Thus, by continually removing plagioclase from contact with the liquid, it is possible, under perfect fractionation conditions to produce an almost pure albitic plagioclase from a liquid which would give a very calcic plagioclase under perfect equilibrium conditions.

Examples of questions on this material that could be asked on an exam

First note that phase diagrams similar to any of the above could be presented on an exam and you could be asked to (a) trace the crystallazation history of any specified composition, (b) determine the temperatures of first melting of any specified composition, (c) determine the composition of all all phases present in any specified composition at a specified temperature, and (c) determine the proportions of all phases present in a specified composition at a specified temperature. A practice exercise for this material can be found by clicking HERE.

Return to EENS 2120 Home Page